The Organization of Sensory Experience into Sensory Spaces and Their Coordination with Physical Space

by Huntley Ingalls

Abstract

Visual, kinesthetic, and tactual sensory fields, visual afterimages, and dreams have spatial characteristics, although physical space is commonly considered to be the only real space. Characteristics of non-physical spaces will be described using the concept of sensory spaces, which was introduced by the French mathematician Henri Poincaré at the beginning of the twentieth century. Poincaré argued that space should not be conceived of as an immutable medium containing objects and events, but as a field of operations that is supported by a variety of processes and operations and the laws by which they succeed one another. The coordination of sensory spaces with physical space will be discussed by approaching the idea of space through the mathematical concept of a manifold--an idea more fundamental than space, which is a type of manifold. This approach facilitates a deeper understanding of the nature of spaces, the ways in which spaces are generated, and how sensory and dream spaces have extension that does not conflict with the extension of physical space.

Visual, tactual, and kinesthetic sensory experiences, visual afterimages, and dreams have distinct spatial characteristics. The relation of these spaces to physical space is a difficult issue in the philosophy of perception. How can sensory images and dreams have spatial features and how can such spaces coexist with physical space, which is commonly assumed to be the only real space? Clear insights into these difficulties are possible through ideas on the nature of space that have been developed by intensive geometrical research in the last 150 years. A favorable ground for pursuing these ideas can be prepared by considering the development of spatial concepts and operations in children or congenitally blind people whose sight has been restored by surgery. This can relieve the constraints of mistaken attitudes and preconceptions about the nature of space that make confusion almost inevitable.

Piaget's research shows that the child's concept of space begins with an almost undifferentiated flux and is built up through several very definite and revealing stages.¹These stages include: the recognition of certain constant combinations of features and patterns; the ability to follow a moving object and recognize the invariance of its form despite its movement; the understanding that an operation, such as hiding an object, can be undone by a second operation; and the child's discovery that he can go from A to B by many paths, and if this is done by any one path he can undo it by many alternate paths. At the end of these stages the child acquires a mental map of the world with a set of permanent places occupied by various objects, one of which is himself from which there is a unique perspective on the world. Later these concepts of space are extended to the refinements of perspective, topological relationships, and geometrical thought. This map pervades perception so intimately that it seems to be an inevitable and necessary feature of experience, and it becomes nearly impossible to question its basic nature.

Congenitally blind people whose sight has been restored by surgery experience great difficulty perceiving three-dimensional space and the relations between objects. They are able to see little at first, and cannot name objects or distinguish between simple objects and shapes. The visual field is a confused pattern of light, dark, colored, and moving visual sensations that bear little resemblance to the visual field of a normal person. They tend to see only the outlines of solid geometrical figures; for example, a sphere appears to be the same as a disk. There is usually a long period of learning before the formerly blind can develop useful vision, and in some cases this is never accomplished. Some give up the attempt and revert to a life of blindness, often after a period of severe emotional distress. Some quickly learn to see rather well; particularly those who are intelligent, active, and who have received a good education while blind. One of these, a man who had been blind from the ages of 10 months to 52 years, enjoyed making things with simple tools and longed for a time when he might see. He tried throughout his life to picture the visual world. When his bandages were removed he did not suddenly see the world of objects as a normal person does. He was able to use his eyes well after a few days and judge distances and sizes accurately if he already knew a particular object by touch, although his sense of vertical distance was much distorted. He was unable to recognize objects that he had not touched and was unable to draw anything he did not already know by touch. His tactual and kinesthetic experience had enabled him to develop a sense of space which, after some effort, could be transferred to his newly acquired vision.²This research confirms the ideas of the French mathematician Henri Poincaré, who wrote in the early twentieth century that isolated sensations cannot provide an individual with a concept of space; rather, this is developed through learning the laws by which these sensations succeed one another, especially in response to the voluntary movements of the individual.³Space is a concept that is abstracted from physical operations, and not a static medium containing objects.

The concept of sensory spaces becomes more accessible through the mathematical idea of a manifold. The concept of a manifold is more general than that of space, which is a subset of manifolds. It enables us to develop basic ideas that are fundamental to the concept of space. Since the idea of a manifold is more abstract and unfamiliar than that of space, we do not approach it with the same ingrained attitudes we have about space. This enables us to bypass some of our preconceptions and permits a fresh and powerful perspective on the concept of space.

Manifolds

The location of any book in a bookcase can be specified by two descriptions; for example, third shelf from the top, eighth book from the left. The location of any bead in a string of beads can be specified by a single description, such as eleventh bead from the left. A manifold is any set of elements such that the location of any element in the set is completely specified when all of n essential descriptions are given. The elements may be objects, operations, or anything specifiable, and the description may be any clearly defined characteristic that is variable, such as distance, brightness, or weight. A manifold is connected if for any two of its elements there exists a chain or path between them such that every element of the path is a member of the manifold. The string of beads is connected; the shelved books are not because there is no path of books between the shelves. A connected manifold is discrete if a passage along a path in the manifold encounters abrupt changes in the varying characteristics; otherwise it is continuous. For example, movement along a string of beads, or through a series of Morse code sounds, encounters sudden changes between the elements. The colors of the spectrum, or the muscular sensations of extending an arm, form a continuous series of gradations into other colors or muscular sensations.

A manifold is one-dimensional if the removal of a single element divides it into two parts such that there is no unbroken path between the parts. A manifold is two-dimensional if the removal of a one-dimensional manifold divides it in a similar manner. It is n-dimensional if it is necessary to remove an (n-I)-dimensional manifold in order to divide it.⁴For example, a succession of musical notes of continuously varying pitch is a one-dimensional manifold, because the removal of a note in the succession makes any passage through it encounter a sudden jump in pitch. If each note can be sounded with both varying pitch and intensity, two descriptions are necessary to specify a sound, and we now have a two-dimensional manifold. The removal of a single note will not cut it into two parts. A one-dimensional manifold must be removed; for example, all notes at middle C in all its intensities. Any path between notes less than and greater than the removed pitch would encounter a sudden jump. Similarly, each note may be varied in tone to form a three-dimensional manifold.⁵

Continuous connected manifolds are very common in ordinary life. The shades of gray between black and white, and the spectrum of weights between two given weights are one-dimensional manifolds.⁶The color continuum is a three-dimensional manifold of hue, saturation, and brightness. A sensory manifold is any manifold that depends on the stimulation of a definite set of sense organs in a particular individual. The sensory manifolds are especially significant for the idea of sensory spaces.

Suppose a person is blindfolded and his skin touched with two pin points. That person can distinguish between the points if there is sufficient spread between them, but when they are brought together they are felt as one point. From any tactual point on the skin there is a path of such points to any other tactual point on the skin; that is, there exists a one-dimensional manifold of tactual points between any two such points on the skin. Now consider a continuous series of such points encircling the arm, and then imagine that they have been removed. All the tactual points have now been divided into two distinct domains, the points that are on the lower arm and those on the rest of the body. All paths between points in these two areas are broken by the series that was removed. It is necessary to remove a one-dimensional manifold of tactual points from the skin in order to divide it into two separate domains of tactual points. The set of all tactual points on the body is a two-dimensional sensory manifold.

Visual manifolds are more complex. The basic visual field is two-dimensional. Visual elements are directly perceived to the right and left and above and below the direction of sight. Objects are also perceived at various distances from the eyes, but this third dimension of the visual field does not have the simple direct quality of the other two. Otherwise, the far side of opaque objects would be visible. The visual elements are assigned distance values according to a complex set of cues, such as muscular accommodation in the eye, hue, brightness, superposition, perspective, and texture-density gradients. The visual elements are distributed in a two-dimensional field, but the cues assign a third characteristic to the elements which makes the visual experience a three-dimensional field. Turning the head or walking generates a continuous family of visual fields which are integrated into a single system. Such activity adds parallax shift, which is an additional distance cue. The family of visual fields arising from the observer's movements and past experiences is integrated into a common manifold. The various descriptions specifying the location of elements in this integrated family of visual fields can always be reduced to three independent descriptions.

The set of feelings that corresponds to the various stages of contraction of a muscle is a one-dimensional manifold. All of the families of muscular sensations are integrated into a common three-dimensional kinesthetic manifold. Two different kinds of sensory manifolds can be combined into a common manifold, and the equivalence values between the two kinds of sensory elements reduce its dimensionality. For example, vision and the handling of objects are coordinated into a visual-kinesthetic manifold. When one reaches for an object, the hand is seen to touch it exactly when the arm is felt to be sufficiently extended. No more than three descriptions, visual or kinesthetic, are needed to specify any element of this system. For example, the location of an object may be described by saying "Walk thirty paces forward, then fifty paces to the left, then look up eight feet."The system is further elaborated by incorporating values from other senses, such as tactual and auditory ones. All the senses are integrated into a three-dimensional general sensory manifold.

Spaces

Connected manifolds are the essence of space. Understanding space through this concept provides a perspective of its basic nature that is free of misleading preconceptions. A space is a connected manifold such that each element has associated with it subsets of elements called neighborhoods. A neighborhood may be thought of loosely as a region of elements having the same general properties as the manifold. For example, any small region on the surface of a sheet of paper is a two-dimensional manifold similar to the two-dimensional manifold of the entire sheet. The elements are called points, and the kind of space depends on the particular axioms that the neighborhoods satisfy. The most familiar spaces, when idealized, satisfy the following conditions:

1. Each point has at least one neighborhood.
2. Any two neighborhoods of the same point have a common subset, which is a neighborhood of that point.
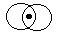
3. If the point y is contained in a neighborhood of the point x, there exists a neighborhood of y, which is a subset of the neighborhood of x.
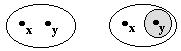
4. For any two distinct points there exist two neighborhoods without common points.

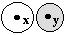
The familiar space of normal life satisfies these conditions. Visual, tactual, and kinesthetic manifolds are less stable,⁷Yet, as they are experienced, they generally satisfy these axioms and are sensory spaces. We have seen that the sensory manifolds are integrated into a more complex manifold of sensations, memories, and abstract manifolds to form a more stable general sensory manifold. This manifold satisfies the axioms more consistently, and it is a space.

The neighborhood axioms outline the connectivity properties of a space, that is, their topology. A metric space is a space in which all pairs of points have a well-defined distance relation.⁸For example, the separation of any two points in the space of a room may be expressed in centimeters.

Consider an extended one-dimensional space whose elements are points of light consecutively ordered by increasing brightness. Each element is uniquely situated in the space by its brightness specification, but it is not necessary to designate a brightness value in order to locate an element uniquely. The nth brightness is also simply the nth element. A position can be associated with every element of the space, and the set of all positions is itself a space. Such second order spaces can be associated with primary spaces of any dimensionality, and the corresponding spaces have identical mathematical characteristics. We can think of such abstract spaces as background for the primary elements. This provides us a powerful basic system as a reference for the primary elements. The background space is abstracted from the primary elements and their relations. They can no more stand alone than the current of a river can flow without water, although it is very expedient to work with the abstract properties of spaces as with purely mathematical hydrodynamics. In the sensory spaces we have an operational awareness of both primary elements and abstract positions. The general sensory manifold is a very complex unity of concrete and abstract spaces.

The operational experiences of ordinary life support a concept of universal space that incorporates all the sensory spaces into a general frame of reference for the familiar world. This is what is thought of as public or physical space. It is the space referenced in describing places, shapes, and locations to other people. Physical space has many advantages over sensory space. It is not centered in the individual. It incorporates a lifetime of experienced sensory spaces and includes the ground of possible sensory spaces that have not been experienced. It is far more consistent and dependable than sensory spaces. Unlike sensory spaces it is homogeneous and isotropic.⁹A very important characteristic of physical space is represented by the postulate that the interval between any two points on a rigid rod remains independent of any motion of the rod, thus enabling consistent measurements.

This line of development is continued in geometry by considering a mathematical space that is an idealization of physical space. It is then possible to study the purely logical properties of this ideal space. While Euclidean geometry appears to represent physical space quite well, mathematicians have constructed other internally consistent spaces. It is possible that some non­Euclidean geometry could be a better model of the physical universe on a cosmological scale. Pure mathematicians even postulate spaces that have no relation to the physical world. They are concerned only with the purely mathematical properties of these ideal constructions.

Physical space and time are commonly thought of as systems of infinitesimal points and instants, but these are concepts of ideal mathematical space and time. Empirical spaces differ from such ideal spaces. A person's vision has a lower limit of acuity, although there is no awareness of the grain of the visual field. The cell assemblies that operate as functional units in the retina, lateral geniculate body, and visual cortex suggests that the sensory fields do not function in the same manner as operations performed on isolated independent points. The operational elements of the sensory fields are not the momentary elementary sensations described by the

structuralists, but are entities such as line segments, corners, or vowels, which reflect the pattern of neural operations. Infinitesimal points and instants are essential concepts in the mathematical analysis of the ideal spaces of geometry and physics, but they are misleading for sensory spaces. Physicists have considered the possibility that even physical space and time are not composed of infinitesimal elements.

Human perceptions do not arise from operations on points of from momentary sensations. An overall structure is built up and abstracted from looser and more expanded elements, from which points and instants can then be constructed. The smallest elements common to the overlapping of the operational units of sensory space define its points. These natural units can then be redefined in terms of points. Thus, the operational priority of the units becomes replaced by the structural priority of points, which misleadingly suggests that points have operational priority. Isolated sensations are not elementary experiences. However, ideal concepts have mistakenly led to the structuralist school of perception.

One of the features most intimately associated with the familiar space of ordinary life is extension. Extension is not the same as distance. Time is not extended in the manner of space, yet it satisfies the conditions of the distance relation. Extension is regarded as such a universal and convincing characteristic of the space of human experience that it is considered to pervade throughout the world and encompass all of existence. This belief is problematic in the philosophy of perception, and makes it necessary to understand the nature of extension and its implications for sensory spaces.

A space is extended if it satisfies the following conditions:

1. All pairs of elements have separation; this is the last of the four neighborhood axioms.
2. All elements exist simultaneously.
3. For any two elements, and any path within the space connecting them, there is a possible operation for traversing that path by a movement that acquires the specifications of each element as it is encountered.

The first condition is the basis of the idea of "here," "there," and "a partness." The second condition is partially the basis for the strong feeling of reality and stability associated with ordinary space. From the vantage point of any part of an extended space, other parts are considered to be there now. The third condition provides the feeling of access, in principle, to all parts of an extended space. These conditions are not only satisfied by physical space, but also by the visual, tactual, and kinesthetic fields and by general sensory space.

A series of objects of continuously increasing weight has separation and simultaneity, but there is no movement from one weight to another that acquires the varying weights. There can be the implied movement that results from changes in attention, but attention is not the same as weight.

Voluntary movements are critical to the development of the concept of extended space because access, in principle, is a fundamental feature of such space. Thus the kinesthetic manifold is a uniquely important subspace of the general sensory manifold. One can directly control and change separate parts of kinesthetic space at will, allowing an ease of direct access to all its parts unmatched by the space of other senses. This feature generates a feeling of being a part of physical space while the other senses provide a feeling of observing it. In kinesthetic perception an object can actually be surrounded by a system of muscular movements. The object then appears to be embedded in a space generated by the movements of the body. This special characteristic of kinesthetic space is incorporated into general sensory space which is then generalized into physical space.

The imagery in dreams that are clear not only satisfies the criteria for space, but also for extended space. Dream spaces are usually extended in three dimensions, with separation, simultaneity, and voluntary movement among the elements. The philosopher H.H. Price described the spatial relations of dream images in vivid language:

If I dream of a tiger, my tiger-image has extension and shape. The dark stripes have spatial relations to the yellow parts, and to each other; the nose has a spatial relation to the tail. Again, the tiger-image as a whole may have spatial relations to another image in my dream, for example to an image resembling a palm tree.¹⁰

It is common in dreams for a person to walk down a path, cross a bridge, see a face, look into a canyon, or approach some object of interest, all of which are spatial experiences in the dream.

Physical space is commonly regarded as a unique medium in which all of existence is embedded. This implies that there can be no extended spaces other than physical space, and hence that dream and sensory spaces are unreal or paradoxical. The imagery in dreams has extension, yet the extension of a dream space cannot be extension in physical space. This paradox is resolved by the realization that space is nothing more than a special kind of organization of elements. A system of elements may be a space under the organization of one domain, but not a space when viewed through the organization of a different domain. The source of a dream space is neural events occurring in physical space, but the geometric relations in dream space are not geometric relations in physical space. A pair of independent radiophoto transmissions occurs in the same space from the viewpoint of the physical world, but there are two image spaces from the viewpoint of the organizations within the signal variations. There is a basis in physical space for the image spaces, but that basis is only a play of signal variations when viewed within the geometry of physical space. What is space from one organizational vantage point may be only a collection of disordered elements from another. Thus it is possible that dreams and drug visions, which are extended spaces, can have an electrochemical basis in the brain and yet be completely unrecognizable as images and spaces from an ordinary physical point of view.

Extension in dreams does not conflict with the extension of physical space, because the elements and operations in dreams are not a recognizable submanifold of physical space. A dream manifold is not merely a simulation of extension. The third dimension supported by perspective and other cues in a painting is a simulation of extension, because there can be no access movement in that dimension except through changes in attention. There is motion in the third dimension in a movie, but there is no way for that motion to be accessible. It is possible to control movement in the third dimension of a mirror, but the source of that control is not within the image space. The image space is extended in a third dimension in accordance with the image behavior, but the source of movement is not in that space. Dream spaces are usually extended in three dimensions, but sometimes only in two, and there is separation, simultaneity, and voluntary movement among the elements. Unlike the case of images in a mirror, in a dream the source of movement is within the space. The visual, tactual, kinesthetic, and general sensory spaces also have genuine extension, and their association with physical space is sufficiently close to support the common attitude that those extensions are somehow part of the extension of physical space. Approaching the concept of space by means of the idea of manifolds clearly shows how it is possible for spaces to exist within spaces, that have no common organization except from a perspective outside the system.

The idea that the familiar extended space of ordinary experience is generated by events and is not a unique and eternal medium in which objects are immersed is startling to common sense. The complex of sensory and physical spaces and their second-order fields, which are ordinarily termed "space," has such a comprehensive framework that it is difficult to show that space is not an immutable medium without implicitly assuming such a medium. Our spatial framework is so constantly present and familiar that when we attempt to imagine it in the absence of operations_, a second order field remains before us and we speak of "empty space." But that space is not truly empty. We have subtly intruded unconscious attitudes into it, which support that space. Thus space is usually construed to be an absolute entity. Liebnitz thought of space as "an order of coexistences"but this idea was two hundred years ahead of its time.

Generation of Sensory Spaces

The mutability of the sensory spaces is more readily apparent. Tactile space is abolished when the body is immersed in water at body temperature and sensation is nullified. When all muscular activity ceases, the kinesthetic space of the moment is nothing more than rather vague attitudes associated with previous muscular experience, and thus it is clear that kinesthetic space is entirely dependent on muscular activity. It becomes clear that the third dimension of visual space is generated, when we perceive the effects of altering basic depth cues by optical instruments or reversed lighting. A different three-dimensional space is then experienced, which may have exaggerated depth, be stretched out, or reversed. When a person first wears new eyeglasses, his visual and kinesthetic coordination are initially upset. The street may look closer than his sensations indicate. His general sensory space is distorted by altered correlations until he is able to learn a new system. The basic two-dimensional field is never absent in the waking state, but the deterioration of acuity toward the edges of the field and the phenomenon of the blind spot reveal that it is generated.

Physical space is less mutable than general sensory space, yet modern physics has shown that physical space is not independent of physical processes. It is distorted under the extreme conditions considered by relativity theory. The geometric properties of physical space are determined by matter and its transformations.

Some sensory spaces have the character of a medium, which is any definite kind of entity distributed in the manner of an extended space. A painting is supported by the medium of paint. The momentary visual field is the medium of visual sensations. Kinesthetic space is not a medium, because its extension is supported not by muscular sensations alone but by those sensations and attitudes about possible muscular sensations. General sensory space is a fabric of sensory media, operations, and attitudes. Physical space is a manifold of physical transformations and their second order spaces. Physical space is not a medium, but our means of conceptualizing it subtly projects it on imaginary media. The visual field is a very strong source of this projection. The tendency to think of physical space as a kind of nothingness which is yet a medium contributes to the confusion felt when we try to imagine the limits of a finite physical universe. None of the higher-order spaces is a medium, because the elements have only mathematical properties.

Spaces have no independent substance of their own. All are dependent in various ways on first-order elements and their relations. The main difference between the spatiality of sensations and that of objects is the degree of refinement to which metrics can be assigned to them. Ideas of physical space are developed by means of experiences with physical entities. As Poincaré observed, the body is a crude measuring instrument, and the instruments that the child owes to nature and those the scientist owes to his ingenuity have the same fundamental basis in the solid body and the light ray.¹¹Physical space has no properties independent of the instruments used to measure it, and to geometrize is to study their properties.

The familiar extended spaces have a smooth contiguity from region to region that appears to be one of their immutable features. Yet the visual field has a lower limit of acuity, although we are not aware of boundaries or gaps between the visual elements. Direct scrutiny of the visual field is incapable of detecting them because they do not exist for that operation, just as one cannot feel the roughness between the grains of sandpaper. Any operation that traverses a set of elements in such a way that gaps are undetectable has a smooth continuity for that operation.¹²A time lapse movie of a door in which each frame shows only a closed door may have gaps in which the door is open, but that will be completely invisible in the film and only a smooth continuous sequence of a closed door will be apparent. Areas that are contiguous in one operation may not be with respect to another. It is possible to construct a set of contiguous areas on a flat surface which are in separate planes situated at various distances when viewed in a stereoscope.

The Pulfrich pendulum illusion is an elegant demonstration of the neural system's ability to generate space. Cover one eye with a dark transparent glass and let a pendulum that consists of a weight and a string several feet long swing in a straight arc perpendicular to the line of sight. When the oscillating bob is viewed with both eyes, it appears to circumscribe an ellipse. The eye that is adapted to the dark takes a longer time to integrate energy and send messages to the brain; hence it sees the bob slightly in the past. The disparity in the effective position of the two eyes generates an ellipse which the bob appears to trace in its swings. The time discrepancy is equivalent to a binocular parallax effect which, for the brain, is exactly as if the bob were really swinging in an ellipse. Julasz's random dot stereograms create another effect that vividly demonstrates the ability of the central nervous system to generate space. These are special pairs of displays constructed with a computer in such a way that each display consists of random dots with no recognizable structure, but when viewed stereoscopically they are perceived as a geometrical structure lying in depth. These spaces are not in the physical optics of the situation, but in the organization of sensory input by the neural system. Such clearly constructed purely synthetic spaces have the same basic quality as the spaces of ordinary experience, and there is a continuous spectrum of spatial experiences between them.

Sensory spaces are not known by direct apperception, but are generated by neural activity coordinated with surrounding physical space. Much of sensation is not experienced at the location of the nerves that support it. This is vividly illustrated by the amputee's experience of the phantom limb. The colors that are induced by the flicker of a strobe seem to be in front of the face, not at the retina or visual cortex. This disparity between the location of a nerve and its sensation indicates that the whole field of a person's sensation is a projection, for there is no difference between the sensation of a nerve stimulated in these unusual ways and one that has been simulated by natural agents.

The topology of the neural network and its events has no necessary resemblance to the topology of the sensory experiences. The physical events in the brain that support sensory or dream space may be highly scattered, but the operations between them give the feeling of contiguity which is spatially convincing. The source of sensory spaces is neural events in physical space, but their geometry is not in physical space.

The union of general sensory space and physical space is the ordinary space experienced in daily life. The familiar world is the union of ordinary space and experience which has location in this space. Dreams and drug deliriums are not in the ordinary external world, but their spaces can be so well developed that the perspective of objects actually changes to correspond with the movements of the observer within them. Hallucinations are false perceptions that appear to be located in ordinary space. Visual afterimages are an interface between visual and physical spaces. They have definite locations in the immediate two-­dimensional visual manifolds, but not in the three-dimensional general sensory space or in physical space.

Conclusion

A manifold is an ordered assemblage of elements each uniquely located by exactly n specific descriptions. Space is a special kind of manifold. Spaces are of many varieties from Poincaré's sensory spaces to ideal mathematical spaces, dream spaces, and hybrid spaces such as general sensory space. Understanding spaces as manifolds clearly shows how spaces can be distorted, and generated, and how sensory and dream spaces can have extension which does not conflict with the extension of physical space.

---

1 J. Piaget, The Origin of Intelligence in the child, Routledge and Kegan Paul, London, 1953. J. Piaget and B Inhelder, The Child's Conception of Space, Routledge and Kegan Paul, London, 1956.

2 M. Von Senden, Space and Sight, trans. P. Heath, Methuen-Free Press, 1960. R.L. Gregory and J.G. Wallace, "Recovery from early blindness: a case study," Exp. Psychol. Soc. Monograph No.2, Cambridge, 1963.

3 Henri Poincaré, Mathematics and Science: Last Essays, Chs. 2 and 3, Dover, New York, 1963.

4 For our purposes, we do not need to consider the problems of higher con nectivities. For example a circle is a one-dimensional manifold of points but two points must be removed to cut it into two parts. Any n dimensional manifold such that the removal of a single n-i dimensional manifold cuts it into two parts is said to be simply connected. The manifolds with which we will be concerned are simply connected.

5 This example is from A. d'Abro, The Evolution of Scientific Thought, Dover, 1950.

6 Given a graded gray wire, the gray and the metal are one-dimensional manifolds, and so is the combination. We need to be mindful of what features we are considering.

7 For example, the visual field is coarser toward the periphery. Another example: two pin pricks on the skin, when very close together, are experienced as a single prick.

8 The distance relation satisfies the following conditions. Let d(P,Q) be the distance between P and Q, then:

d(P,P) = 0, d(P,Q) > 0 if P ≠ Q

d(P,Q) = d(Q,P)

d(P,Q) + d(Q,R) > d(P,R)

9 For example, the immediate visual field is not homogeneous. Visual acuity at the periphery of the visual field is much lower than at the center. The kinesthetic space of arm movements is not isotropic. Extending an arm feels different from moving it sideways.

10 H.H. Price, "Survival and the idea of 'another world"', Proc. Soc. Psychical Research, 1953, pp. 11-12.

11 Poincaré,Mathematics and Science: Last Essays, Ch. 3.

12 Although these operations can generate contiguity and extension in a visual or kinesthetic field, these features of a field are not sufficient for the perceptual unity which is present in consciousness, and the binding problem is not solved.